Bayes, Thomas

Genealogy

Clay (1895) records in the Familiae Minorum Gentium that the Bayes family trade, carried out in Sheffield in England, was cutlery (perhaps more generally steel working). Records suggest that the family was a respected one with several of Thomas’s seventeenth-century male ancestors being Masters of the Company of Cutlers of Hallamshire.

Thomas Bayes came from staunchly Protestant stock. His great-uncle, the clergyman Samuel Bayes, was ejected from his living by the Act of Uniformity, passed by the anti-puritan parliament in 1662. In terms of this Act, all clergymen who refused to follow the rubric laid down in the Book of Common Prayer and Administration of the Sacraments lost all spiritual promotions. In Samuel’s case this resulted in his having to move several times before ending up in Sankey.

Samuel’s brother, Joshua, became a cutler in Sheffield and was prominent among Nonconformists in that town. He and his wife Sarah (née Pearson) had seven children. The second eldest son, also Joshua, married Anne Carpenter and in turn had seven children, the eldest of whom was Thomas.

Joshua (II) attended Richard Frankland’s dissenting academy (such academies were essentially institutions of university status, but did not demand the profession of faith required of students of Cambridge or Oxford). In 1694 he was ordained in London along with six other candidates for the ministry at the meeting-house of Dr Samuel Annesley (whose daughter Susanna became the mother of John and Charles Wesley). After his ordination Joshua moved to Bovingdon, Hemel Hempstead, Hertfordshire, where he officiated at the Box Lane Chapel. It is possible that Thomas was born here, for Urwick records that Joshua stayed at Box Lane for about eleven years.

In the early eighteenth century Joshua returned to London, where he assisted John Sheffield at St Thomas’s, Southwark. In the 1720s he became the full-time minister at the Leather Lane meeting-house in Hatton Garden, where he stayed until 1746, the year of his death.

Joshua was well regarded by his fellow Nonconformists in the capital. He served on the general committee of the Body of Protestant Dissenting Ministers of the Three Denominations in and about the cities of London and Westminster, on a number of occasions as chairman. Further, on the death of Matthew Henry, Joshua was tasked with the completion of the Commentary on Galations. Described as a man of “good learning and abilities’’ (Wilson 1814: 399), who took pains in the preparation of his sermons, Joshua was perhaps not altogether a popular preacher.

Education

Where Thomas Bayes received his early education is unknown; it might well have been at one of the dissenting academies in London, one of the possibilities being that run by John Ward. However his name is to be found in the annals of Edinburgh University. In 1719 he was admitted to the use of the university library, the certificate being signed by James Gregory. This James was the brother of his immediate predecessor, David, in the chair of mathematics, and the nephew of the more famous James, who was the first substantive Professor of Mathematics in the university.

There is no evidence that Bayes studied mathematics during his time at university; however, he is known to have studied logic under Colin Drummond. James (I) Gregory had introduced Newton’s fluxional calculus into the Edinburgh curriculum while he was there, and it is possible that instruction in this calculus was continued by his nephews.

Bayes, like many of his coevals who intended to become Nonconformist ministers, did not take the M.A. degree, and he consequently left Edinburgh licensed but not ordained. Moving to London, he became assistant to his father at the Leather Lane Chapel for a few years before moving to Tunbridge Wells in the early 1730s.

Ministry

By this time Tunbridge Wells, on account of its revivifying springs, had grown from its somewhat humble beginnings to a most fashionable watering place. Those who visited there to “take the waters” during the season, were anxious to take care not only of their physical well-being but also of their spiritual needs. Denominations of various forms were catered for, and among these was the (English) Presbyterian, whose adherents had a meeting-house on Mount Sion. Bayes was to remain as minister at this chapel for more than twenty years.

Successor, though perhaps not immediate, to John Archer (who in turn had followed Humphrey Ditton), Bayes was followed by William Johnson. But the decline of Presbyterianism set in, and over the next few centuries the chapel saw sporadic use by other Protestant sects with intermittent periods of disuse. Today, with exterior still suitably plain but interior greatly altered, the meeting-house is a commercial establishment.

Bayes could of course not escape the notables who visited Tunbridge Wells from time to time. In 1746 William Whiston (Newton’s successor in the Lucasian chair at Cambridge, from which position he was removed for Arianism in 1710) visited Tunbridge Wells, and he noted in his diary that he had breakfasted with Bayes on a specific occasion. Whiston and Ditton had collaborated on the problem of the determination of the longitude, and it was no doubt natural for Whiston to visit one who, like Ditton, exhibited both scientific and theological interests. Further, when he moved to London on leaving Cambridge Whiston occupied a house very near the Bayes residence, and Thomas may well have had early knowledge of the older savant.

Another visitor to Tunbridge Wells was Philip, Earl Stanhope. That Bayes and Stanhope were acquainted is evidenced by papers in the Stanhope Collection in the Kent County Archives in Maidstone. These documents, found and discussed by Bellhouse, suggest that Bayes was used by Stanhope as a mathematical advisor or referee. The Collection contains some work by Bayes in his own hand, as well as letters referring to mathematical work by Patrick Murdoch and Bayes’s comments thereon.

It seems that Bayes resigned from his ministry in the early 1750s, although he probably stayed in Tunbridge Wells until his death in 1761.

Works

Only two works were published by Bayes during his lifetime. The first of these, Divine Benevolence, Or, An Attempt to prove that the Principal End of the Divine Providence and Government is the Happiness of his Creatures, was published in 1731. Here Bayes argued that God is motivated by benevolence in all His actions and dealings with His creatures. The argument was presented in some measure as a response to a tract by John Balguy who presented Divine Rectitude as the motivating and guiding principle. Henry Grove in turn later suggested the fundamental place of Divine Wisdom.

The second work was An Introduction to the Doctrine of Fluxions, published in 1736. Although published anonymously, there seems little doubt that this was indeed by Bayes, and it was written in at least partial response to Bishop George Berkeley’s The Analyst; or, a Discourse Addressed to an Infidel Mathematician (1734). Berkeley criticized the methods of both the fluxionary and differential calculi and the ontological status of the objects considered therein. In his response Bayes essentially ignored the theological points raised by Berkeley, confining himself to consideration of the logical analysis, rather than the methods, of the theory of prime and ultimate ratios (crudely speaking, these ratios are analogous to the modern right and left derivatives). The tract is written in a logical way, with postulates, definitions, axioms and propositions following one another in a manner that would not seem foreign to a modern mathematician. One sees here too an approach to the theory of limits that has much to be said for it.

In 1764, a few years after Bayes’s death, Richard Price communicated two papers by him to the Royal Society, these being published in the Philosophical Transactions for 1764. The first of these papers dealt with the well-known Stirling-de Moivre series expansion of log x!, viz.

log x! = (1/2) log c + ( x+(1/2)) log x − S,

where  and

S = 

Bayes noted that the series failed to converge for any value of x. The failure for x =1 had been pointed out by Euler a few years before Bayes died, but there is no evidence to suggest that Bayes was acquainted with Euler’s work.

The work for which Bayes is remembered, however, is An Essay towards solving a Problem in the Doctrine of Chances. Here Bayes proposed the following problem:

Given the number of times in which an unknown event has
happened and failed. Required the chance that the probability
of its happening in a single trial lies somewhere between any
two degrees of probability that can be named.

In modern notation Bayes’s solution, given in his tenth proposition, runs as follows: for any x₁ and x₂ with 0 ≤ x₁ ≤ x₂ ≤ 1,

P[x₁ < x₂|p successes and q failures in p + q trials]

= 

where x denotes the prior probability. Bayes’s own version was given geometrically, using areas, as a ratio of series.

In its most commonly found form the result known as Bayes’s Theorem is usually given as follows:

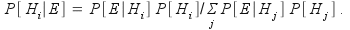
This is essentially the form in which the result was given by Laplace in 1774 in apparent ignorance of Bayes’s paper.

Bayes’s retrospective result was used in the appendix added by Price to investigate the probability that an event known to have occurred a number of times would occur in the future.

A second paper, in which the Rules given in the Essay were more closely examined, was published in the subsequent volume of the Philosophical Transactions. Partly by Price, this article gave tighter bounds on the probability discussed in the Essay, the amended Rules to be used when p or q were large.

Death

On the 7^th April 1761 Bayes died. His body was taken to London and interred in the family vault in Bunhill Fields Burial Ground in Moorgate. The vault still remains, though successive restorations have resulted in some inaccuracies appearing in the inscriptions. A pleasing addition, however, is the recording on one of the surfaces that

In recognition of Thomas Bayes’s important work
in probability this vault was restored in 1969 with
contributions received from statisticians throughout
the world.

Works by Thomas Bayes

1. Divine Benevolence, Or, An Attempt to prove that the Principal End of the Divine Providence and Government is the Happiness of his Creatures. Being an answer to a Pamphlet, entitled: ‘Divine Rectitude: or An Inquiry concerning the Moral Perfections of the Deity’. With a Refutation of the Notions therein advanced concerning Beauty and Order, the Reason of Punishment, and the Necessity of a State of Trial antecedent to perfect Happiness. (1731). London: John Noon.
2. An Introduction to the Doctrine of Fluxions, and Defence of the Mathematicians against the Objections of the Author of the Analyst, so far as they are designed to affect their general Methods of Reasoning. (1736). London: John Noon.
3. “A letter from the late Reverend Mr. Thomas Bayes, F.R.S. to Mr. John Canton, M.A. F.R.S.’’ Philosophical Transactions (1763, published 1764) 53: 269-325.
4. “An Essay towards solving a Problem in the Doctrine of Chances. By the late Rev. Mr. Bayes, F.R.S. communicated by Mr. Price, in a letter to John Canton, A.M. F.R.S.’’ Philosophical Transactions (1763, published 1764) 53: 370-418.
5. “A Demonstration of the Second Rule in the Essay towards the Solution of a Problem in the Doctrine of Chances, published in the Philosophical Transactions, vol. LIII. Communicated by the Rev. Mr. Price, in a Letter to Mr. John Canton, M.A. and F.R.S.’’ Philosophical Transactions (1764, published 1765) 54: 296-325.